Discover the Fascinating World of Probability with the Binomial Distribution and Pascal's Triangle

Do you want to understand the underlying principles of probability and how it affects various scenarios in our daily lives? Look no further! In this article, we will delve into the intriguing world of probability, specifically focusing on the binomial distribution and its close relationship with Pascal's Triangle.

Understanding Probability

Probability is a branch of mathematics that deals with the likelihood of events occurring. It allows us to quantify uncertainty and predict the chances of different outcomes. Probability theory has a wide range of applications, from predicting weather patterns to analyzing stock market trends.

At its core, probability is based on the concept of experimentation and observing results. The outcome of an experiment can be classified as a success or a failure, and the probability of success is denoted by the letter 'p.' Conversely, the probability of failure is represented by 'q' (p + q = 1).

Probability With The Binomial Distribution And Pascal's Triangle: A Key Idea In Statistics

by Scott Hartshorn(Kindle Edition)

4.7 out of 5

Language	:	English
File size	:	3027 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Print length	:	65 pages
Lending	:	Enabled
X-Ray for textbooks	:	Enabled

FREE

The Binomial Distribution

The binomial distribution is a probability distribution model that calculates the probability of obtaining a specific number of successes in a fixed number of trials. It is widely used in various fields, including statistics, genetics, and quality control.

Imagine conducting a series of coin flips. Each flip has two possible outcomes - heads or tails. The probability of getting heads in a single flip is denoted as 'p,' and the probability of getting tails is 'q.'

If we flip the coin 'n' times, we can calculate the probability of obtaining 'x' heads using the binomial distribution formula:

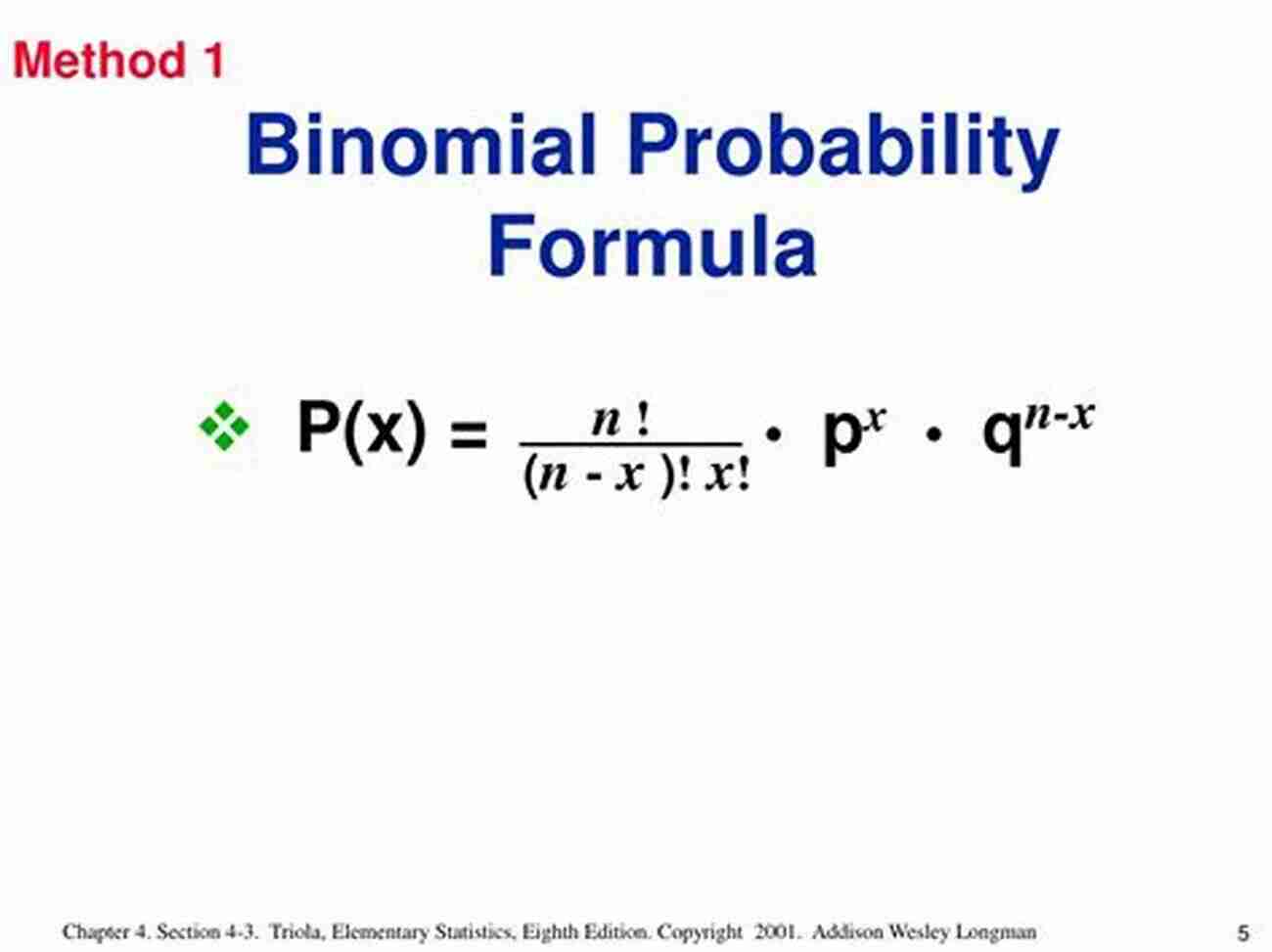
Here, 'C(n, x)' represents the binomial coefficient, which can be determined using Pascal's Triangle.

Pascal's Triangle

Pascal's Triangle is a triangular arrangement of numbers that holds deep connections with various mathematical properties, including the binomial coefficients in the binomial distribution formula.

The first row of Pascal's Triangle consists of the number 1, and each subsequent row is formed by adding the elements diagonally above. The triangle follows the rule that each number is the sum of the two numbers directly above it.

Let's take a closer look at a section of Pascal's Triangle:

1 1 1 1 2 1 1 3 3 1 1 4 6 4 1

The number '1' represents the binomial coefficient when there is only one way to choose or arrange the items. For example, there is only one way to choose all heads or all tails when flipping a coin.

The numbers in the subsequent rows of Pascal's Triangle represent the number of ways to choose or arrange items when there are more possibilities. For instance, there are three ways to get two heads and one tail when flipping a coin three times (HH, HT, TH).

Practical Applications

The binomial distribution and Pascal's Triangle find application in various real-world scenarios:

• Evaluating the effectiveness of a new drug: The binomial distribution can help determine the probability of a certain number of individuals responding positively to a new medication.
• Predicting election outcomes: By using historical data and the binomial distribution, analysts can calculate the probability of a candidate winning based on the number of votes.
• Quality control in manufacturing: The binomial distribution can assess the likelihood of a specific number of defective products in a production line, helping identify areas for improvement.
• Weather forecasting: Statistical models based on the binomial distribution aid in predicting the occurrence of certain weather patterns, such as rain or snow.

Probability, the binomial distribution, and Pascal's Triangle are fascinating tools used to study the likelihood of different outcomes in various scenarios. By understanding these concepts, you gain a deeper appreciation for statistics and their applications. Whether you're interested in making data-driven decisions or simply intrigued by the math behind the world around you, exploring probability will undoubtedly broaden your knowledge and perspective.

So, go ahead and delve into the exciting field of probability. Remember that even the most uncertain situations can be analyzed and understood with the help of mathematics!

Probability With The Binomial Distribution And Pascal's Triangle: A Key Idea In Statistics

by Scott Hartshorn(Kindle Edition)

4.7 out of 5

Language	:	English
File size	:	3027 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Print length	:	65 pages
Lending	:	Enabled
X-Ray for textbooks	:	Enabled

FREE

What Is The Binomial Distribution?

The binomial distribution is one of the key ideas in statistics.  It calculates the probability of getting a certain number of an outcome, for instance you can use it to calculate the probability of rolling five 6's out of 20 dice rolled.  The binomial distribution finds applications in things such as predicting outcomes from elections, in gambling, and even on the game "Plinko" on the television game show "The Price Is Right"

How Is The Binomial Theorem Explained In This Book?

This book walks through how the binomial distribution works in a step by step fashion, starting with some simple flips of a coin, and building up to examples that have uneven probability, and examples where you need to calculate the binomial coefficient over a range of numbers.  I.e.  to calculate the odds of winning at least 51 hands of blackjack out of 100 played.

The first several examples are explained using Pascal's triangle, since it gives a good visualization of the probability of different binomial coefficients.  Later problems give examples using the binomial equation, since it is more versatile.

Other Key Topics In This Book

• Multinomial Equation - If you need to calculate the probability distribution for more than two events, you need the multinomial distribution, not the binomial distribution
  
• The Normal Approximation - The binomial distribution is great, but sometimes you need an answer with less calculation.  This shows how to get a very good answer using the normal curve, provided you have a sufficient number of events